What is venturimeter:-
“It is a device used to measure the speed and flow rate or discharge of fluid through a pipe.” Venturimeter is work on Bernoulli’s equation.
Its basic principle also depend on Bernoulli’s equation and continuity equation. Velocity increases pressure decreases.
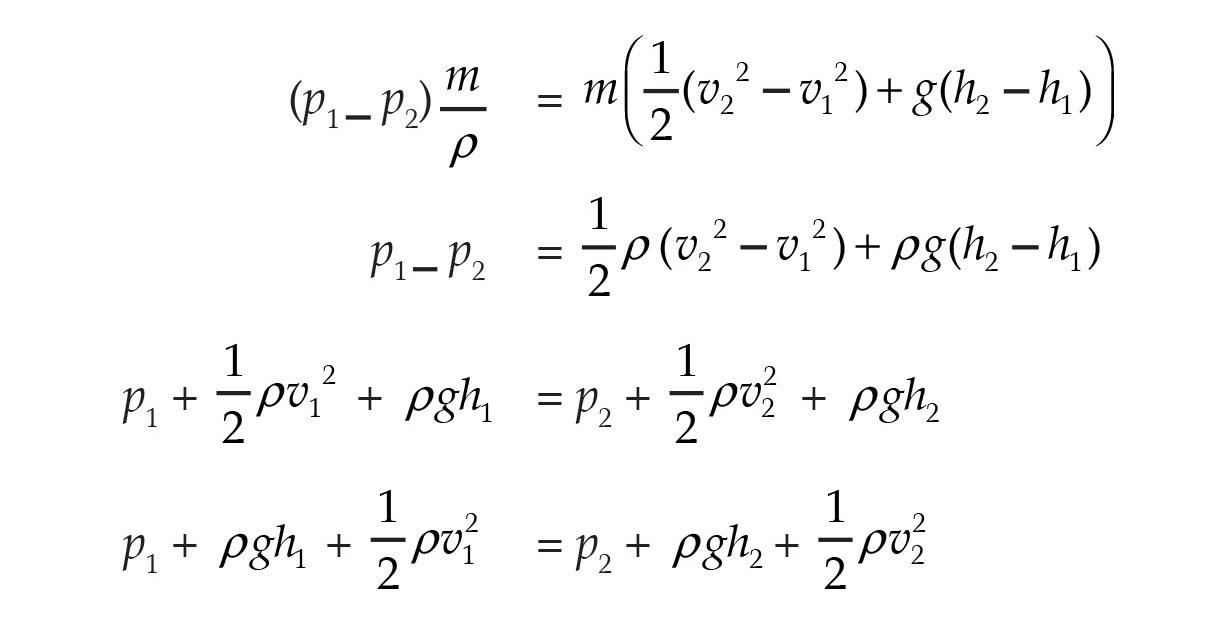
Aplikasi Bernoulli – Venturimeter Dengan Manometer Untuk mencari pembuktian dari rumus diatas, mula-mula kita gambar terlebih dahulu, Mencari P 2 – P 1 untuk membantu mencari pembuktian dari rumus. Itulah yang dapat kami bagikan terkait contoh soal venturimeter dengan manometer beserta pembahasan. Admin dari blog Barisan Contoh 2019 juga mengumpulkan gambar-gambar lainnya terkait contoh soal venturimeter dengan manometer beserta pembahasan dibawah ini. Hanya saja dalam venturimeter ini ada tabung U yang berisi raksa, perhatikan gambar 1. Gambar 1: Venturimeter dengan manometer Kita akan menentukan kelajuan aliran v 1 dan v 2 yang dinyatakan dalam luas penampang pipa dan perbedan ketinggian cairan, h dalam kedua pipa vertikal.
- Jadi venturimeter pada asas bernoulli ini terbagi 2, yaitu: 1. Venturimeter terbuka. Venturimeter tertutup. Rumus venturimeter terbuka: V = akar dari 2gh dibagi (A1/A2)kuadrat - 1.
- Makalah venturimeter fisika kelas XI Percobaan Venturimeter.
Simple meaning is “When cross sectional area of the flow is reduces it creates pressure difference between the different areas of flow. This difference in pressure is measured with the help of manometer and helps in determining rate of fluid flow or other discharge from the pipe line.”
History:- The principle of venture meter is firstly developed by G.B. Venturi in 1797 but this principle comes into consideration with the help of C. Herschel in 1887.

Main parts of Venturimeter:-
1. Converging part
2. Throat

3. Diverging Part

Converging part
It is starting section of venturimeter which attached at inlet pipe. The cross sectional area of this cone starts to decrease and the converging angle is 20 degree. Its length is 2.7(D-d). Here (D) is the diameter of inlet section and (d) is the diameter of throat. Other end of converging is attached with throat.
Rumus Pada Venturimeter
Throat
Penurunan Rumus Venturimeter
Throat is middle portion of venturimeter and its cross sectional area is too small. At this point pressure is decreases and velocity is increases. One end is connected with converging part and other end is attached with diverging part. Diameter of throat is ¼ to ¾ of the diameter of the inlet pipe, but mostly it is ½ of the diameter of the pipe.
Diverging part
Diverging part is last part of venturimeter and its cross sectional area is increases continually. Angle of diverging part is 5 to 15 degree. Its cross sectional area continuously increases. One end is connected to throat and other end is connected to outlet pipe.The main reason behind the low diverging angle is to avoid the formation of eddies because flow separation and eddies formation will results in large amount of loss in energy
Working:-
· Venturimeter is work on Bernoulli’s equation and its simple principle is when velocity increases pressure decreases.
· Cross sectional area of throat section is smaller than inlet section due to this the velocity of flow at throat section is higher than velocity at inlet section, this happen according to continuity equation.

· The increases in velocity at the throat result in decreases in pressure at this section , due to this pressure difference is developed between inlet valve and throat of the venturimeter.
· This difference in pressure is measured by manometer by placing this between the inlet section and throat.
· Using pressure difference value we can easily calculate flow rate through the pipe.
Expression for the rate of flow through venturimeter:-
Let d1, p1, v1 & a1, are the diameter at the inlet, pressure at the inlet, velocity at the inlet and area at the cross section 1.
And d2, p2, v2 and a2 are the corresponding values at section 2.
Applying bernoulli’s equation at sections 1 and 2
As the pipe is horizontal, so z1 = z2
Therefore
(P1 – P2)/ρg is the difference of pressure heads at section 1 and 2 and it is equal to h. so
Substituting this value of h in equation (1), we get
Now applying continuity equation at section 1 and 2
Substituting this value of v1 in equation (2) and solving, we get
Discharge
Substituting value of v2 in above equation
Q is the theoretical discharge under ideal conditions. Actual discharge will be less than the theoretical discharge. The actual discharge is given by the formula
Rumus Kecepatan Venturimeter
Where Cd is the coefficient of venturimeter and its value is less than 1.
Author:-
Gopinath Murthi
